Modelling plant hydraulics within the QUINCY-SIMPHONY framework
Physical principles
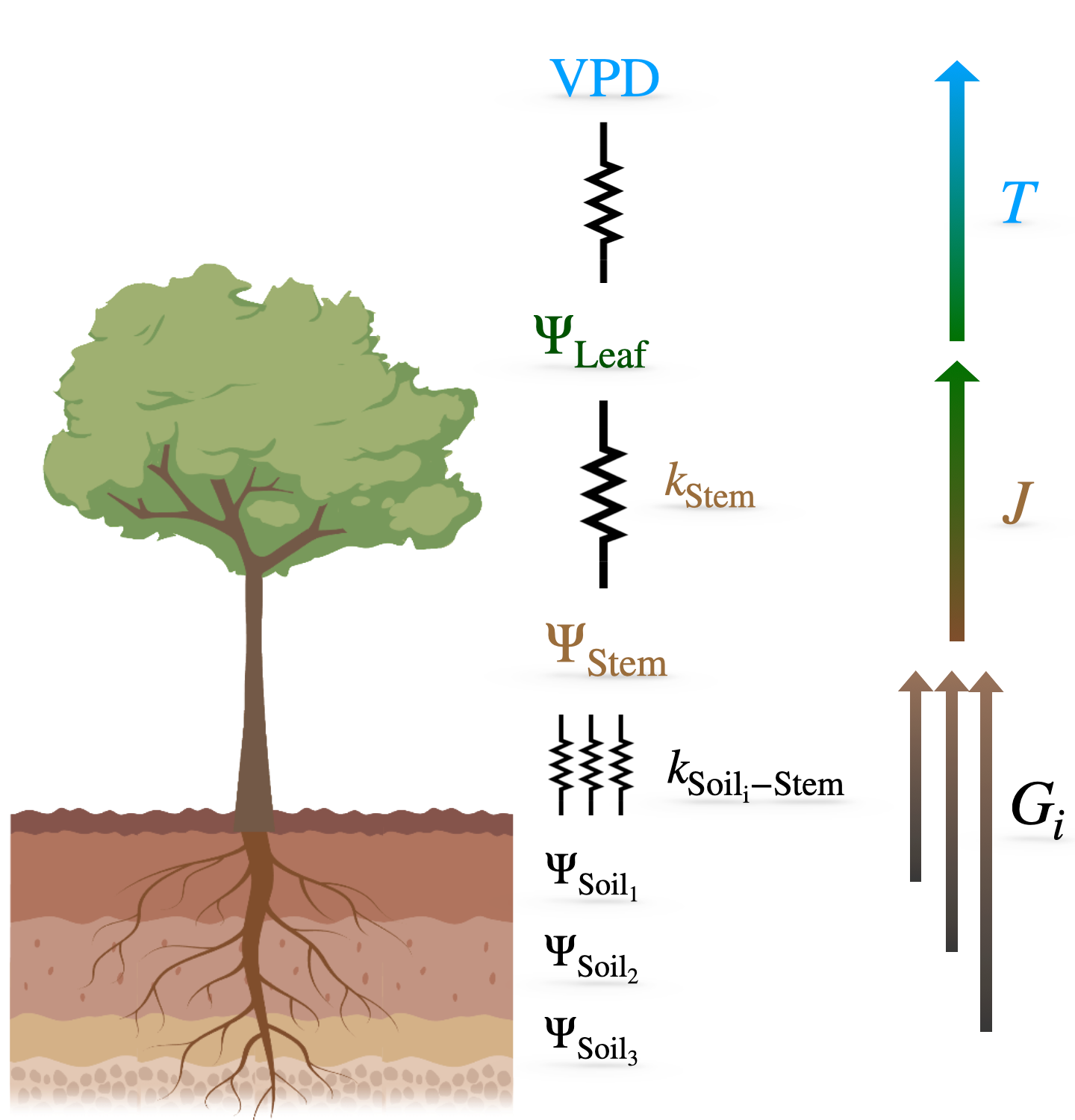
Fundamentally, plant hydraulics can be expressed via a system of two or more coupled mass balance equations. Here, we follow the approach based on Xu et al. (2016) and define the change in water content of accumulated across all leaves and the botten of the stem as:
- $ \Gamma_\mathrm{Leaf}\cdot \frac{d\Psi_{\mathrm{Leaf}}}{dt} - J + T_\mathrm{Leaf} = 0 \label{eqn:psi_L_tree_base}$
- $ \Gamma_{\mathrm{Stem}} \cdot \mathrm{Huber} \cdot H \cdot \frac{d\Psi_{\mathrm{Stem_B}}}{dt} + J - G + T_\mathrm{Stem} = 0$
$\Psi_\mathrm{Leaf}$ is the average water potential a leaf in the canopy and $\Psi_\mathrm{Stem_B}$ is the stem water potential at the bottom of the stem close to the ground.
The fluxes are: Transpiration $T_\mathrm{Leaf}$, Sapflow $J$, Stem water loss $T_\mathrm{Stem}$ and Groundflow $G$.
Transpiration $T_\mathrm{Leaf}$
We estimate transpiration by multiplying stomatal conductance $g_s$ with vapor pressure deficit $\mathrm{VPD}$:- $ T_\mathrm{Leaf}\left(\Psi_\mathrm{Leaf}\right) = g_s\left(\Psi_\mathrm{Leaf} \right) \cdot \mathrm{VPD}$
- $ g_s\left(\Psi_\mathrm{Leaf} \right) = g_0 + \beta\left(\Psi_\mathrm{Leaf} \right) \cdot \left( 1 + \frac{g_1}{\sqrt{\mathrm{VPD}}} \right) \frac{A}{c_a}$
We use a Gompertz function to describe the relationship between $\beta$ and $\Psi_\mathrm{Leaf}$:
- $ \beta\left(\Psi_\mathrm{Leaf}\right) = \exp{\left(-\exp{\left(-s_\mathrm{close,50}(\Psi_\mathrm{Leaf}- \Psi_\mathrm{close,50})\right)}\right)}$
with $\Psi_\mathrm{close,50}$ representing the leaf water potential at which 50\% of stomatal closure is induced, and $s_\mathrm{close,50}$ a shape parameter.
Sapflow $J$
Sapflow is estimated as:
- $J =\sum^{\Psi_\mathrm{Leaf}}_{\substack{\Psi = \Psi_{\mathrm{Stem}_B} \\ \Delta\Psi} } K_{\mathrm{xyl}}(\Psi) \cdot \left(\Delta\Psi - {\Psi_H}_n\right) $
with $\Delta\psi = \frac{\psi_\mathrm{Leaf}-\psi_{\mathrm{Stem}_B}}{n}$ and $ {\psi_H}(n) = \rho \cdot g \cdot \frac{H}{n} $ and $n$ number of stem segments and $H$ is the canopy height. Xylem hydraulic conductivity $K$ is estimated using a Weibull relationship:
- $ K_{\mathrm{xyl}}(\psi) = K_{\mathrm{xyl, max}} \cdot \exp{\left(-\left( {\frac{-\psi}{b}} \right)^c\right)} $
with $K_\mathrm{xyl, max}$: maximum/saturated xylem hydraulic conductivity and $b$ and $c$ are shape parameters that can be estimated with $\psi_{50}$ and $\psi_{88}$
Stem water loss $T_\mathrm{Stem}$
We simulate stem water loss through the bark similar to transpiration $T_\mathrm{Leaf}$
- $ T_\mathrm{Stem} = g_\mathrm{Stem} \cdot \mathrm{VPD} $
Groundflow $G$
The water flow $G$ from the soil to the stem is estimated as sum through the individual soil layers $i$:
- $ G = \sum_i G _i = \sum_i f_i \cdot k_{\mathrm{soil},i} \cdot\left(\Psi_{\mathrm{soil},i}- \Psi_{\mathrm{Stem}_G} - \rho \cdot g \cdot d_{\mathrm{soil},i} \right) $
Parameters
| Parameter | Description | Unit | Parameter | Description | Unit |
|---|---|---|---|---|---|
| $K_\mathrm{xyl,max}$ | Maximum xylem hydraulic conductivity | $\mathrm{kg m^{-1} s^{-1} MPa^{-1}}$ | $\Gamma_\mathrm{Stem}$ | Stem hydraulic capacitance | $\mathrm{kg m^{-3} MPa^{-1}}$ |
| $\Gamma_\mathrm{Leaf}$ | Leaf hydraulic capacitance | $\mathrm{kg m^{-2} MPa^{-1}}$ | $\Psi_\mathrm{Leaf, close}$ | Leaf water pot. at 50% stom. clos. | $\mathrm{MPa}$ |
| $g_0$ | Minimal stom. conductance | $\mathrm{mmol m^{-2} s^{-1}}$ | $g_\mathrm{stem}$ | Minimal stem conductance | $\mathrm{mmol m^{-2} s^{-1}}$ |
| $g_1$ | Stom conductance parameter | $-$ | $\mathrm{Huber}$ | Ratio of sapwood area to leaf area | $-$ |
| $\mathrm{Root}_\beta$ | Root distribution (Jackson et al. xxx) | $-$ | $\mathrm{pore_{size}}$ | Van Genuchten soil water parameter | $-$ |
| $\mathrm{log_{10}}k_\mathrm{soil,sat}$ | Log10 of sat soil hydraulic conductivity | $\mathrm{m s^{-1}}$ | $\theta_r$ | Residual water content | $-$ |
Parameter Settings
Explore the effects of changing these parameters on $\Psi_\mathrm{Leaf}$ and $\Psi_\mathrm{Stem}$ and derived state such as stomatal conductance $g_s$.